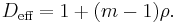Design effect

In statistics, the design effect (or estimates of unit variance) is an adjustment used in some kinds of studies, such as cluster randomised trials, to allow for the design structure. The adjustment inflates the variance of parameter estimates, and therefore their standard errors, which is necessary to allow for correlations among clusters of observations.[1][2] It is similar to the variance inflation factor and is used in sample size calculations.[3] The term was introduced by Leslie Kish in 1965.[4]

Definition

For a cluster randomised trial with m observations in each cluster and intra-cluster correlation of \rho, the design effect. Deff, is given by:[5]

D_\text{eff} = 1 %2B (m - 1) \rho .

Formally, the design effect is the ratio of two theoretical variances for an estimator:[4][6]

  • the actual variance for a given sampling design;
  • the variance assuming the same sample size, but using simple random sampling without replacement.

References

  1. ^ Alexander K. Rowe, Marcel Lama, Faustin Onikpo and Michael S. Deming (2002). "Design effects and intraclass correlation coefficients from a health facility cluster survey in Benin". International Journal for Quality in Health Care 14 (6): 521–523. http://intqhc.oxfordjournals.org/cgi/content/full/14/6/521. 
  2. ^ "Glossary - NCES Statistical Standards". http://nces.ed.gov/statprog/2002/glossary.asp. 
  3. ^ Heo, Moonseong; Kim, Yongman; Xue, Xiaonan; Kim, Mimi Y. (2010). "Sample size requirement to detect an intervention effect at the end of follow-up in a longitudinal cluster randomized trial". Statistics in Medicine 29 (3): 382–390. http://www3.interscience.wiley.com/journal/123212319/abstract. 
  4. ^ a b Kish, Leslie (1965). Survey Sampling. New York: John Wiley & Sons, Inc.. ISBN 0471109495. 
  5. ^ Bland, M (2005), "Cluster randomised trials in the medical literature", Notes for talks, York Univ
  6. ^ Everitt, B.S. (2002) The Cambridge Dictionary of Statistics, 2nd Edition. CUP. ISBN 0-521-81099-x